Spacecraft.CardinalPoints(CelestialObject) Method

Top

Description

Returns the times when the Spacecraft is at each of the cardinal points. The cardinal point calculations are based on the alpha angle, which is the angle between the Spacecraft's position and the target CelestialObjects's position with respect to the Spacecraft's central body when both vectors are projected onto the Spacecraft's orbit plane. Note that the target CelestialObject is modeled as a point.

The mathematical definition of the alpha angle is:

Below is a diagram depicting how the alpha angle is defined:

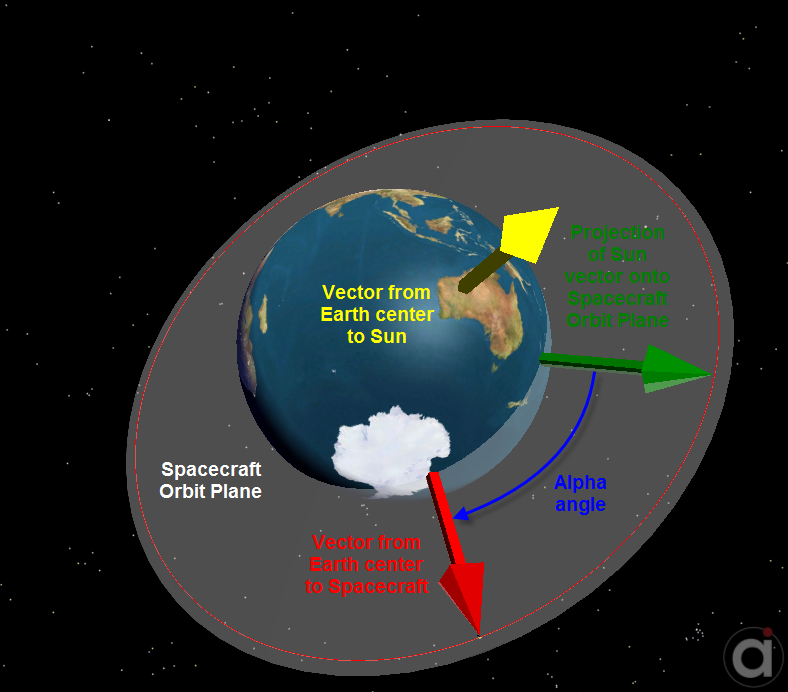
A visual representation of the Alpha angle.

Timing Precision Mode

This page describes functionality in millisecond timing precision mode. Millisecond timing precision mode is deprecated and will be removed in a future release. We recommend that you migrate your Mission Plans to nanosecond timing precision mode.

Click here to see the documentation for this object in nanosecond timing precision mode.

Note: This is an interval method. See the Interval Methods page for more information.

Note: This method has a state. See the Methods and Properties with State page for more information.

Method Signature

Spacecraft.CardinalPoints(

CelestialObject target)

Arguments

target
Description:	The target CelestialObject to be used in the CardinalPoint calculations. If no CelestialObject is specified, the Sun will be used.

Return Value

Type:

string

Returns a string containing the cardinal point and the time when the calling Spacecraft is at that point. The different cardinal points are:

- Noon (alpha angle = 0)

- Dusk (alpha angle = 90)

- Midnight (alpha angle = 180)

- Dawn (alpha angle = 270)

Syntax

myString1 = mySpacecraft1.CardinalPoints(myCelestialObject1);